
Using a mathematical model for the intracellular kinetics of influenza A virus replication in vitro to asses current antivirals
Graduate student of Dr. Beauchemin
Ryerson University
September 2016 - current
This section will be updated soon.

Graduate student of Dr. Beauchemin
Ryerson University
September 2016 - current
This section will be updated soon.

August 24, 2017
Acknowledgment in an article published in PLOS one by Dr. Laura E. Liao.
Graduate student of Dr. Beauchemin
Ryerson University
September 2014 - August 2016
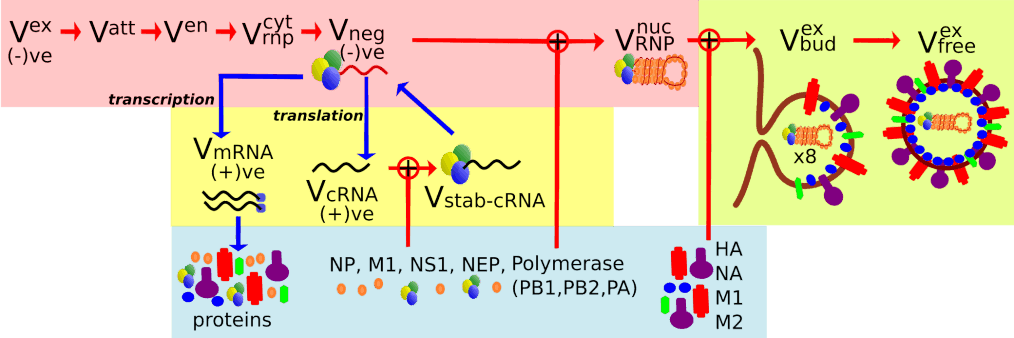
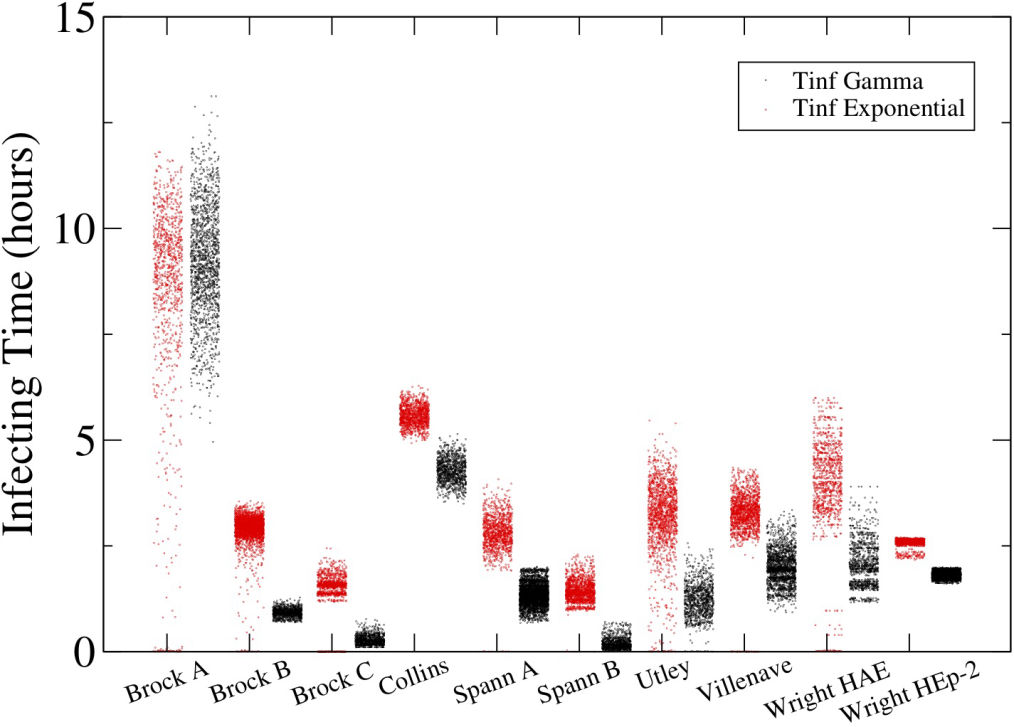
In the last twenty years, mathematical modelling (MM) has been notably used to capture the infection kinetics of many infectious diseases as it allows insights into the basic biology, infection kinetics, and the mechanisms and efficacy of treatment modalities. MMs of influenza A virus (IAV) infection usually represent the process of virus replication within a cell as a ‘black box’ term for viral production. The simplification is appropriate when we are not interested in the microscopic details of infection. Recently though, MMs have begun to account for the kinetics of intracellular IAV replication. Herein, we examine the MM by Heldt et al., which is able to capture kinetics of IAV infection. It however, does so by adjusting parameters of the MM to various events in the infection process. We developed a robust, yet concise, MM for the intracellular kinetics of influenza A virus infection in vitro with a consistent set of parameters. We use attachment, fusion and RNA data gathered from literature sources to validate our simplified MM and match known infection kinetics consistent throughout infection.
This thesis represents the fulfillment of a requirement in order to obtain an MSc degree.
Thesis student for Dr. Beauchemin
Ryerson University
September 2012 - April 2013
The respiratory syncytial virus (RSV) is a common virus of the paramyxoviridae family which causes cold-like symptoms and bronchitis in adults. Currently there exists no mathematical model which describes the kinetics of an infection with RSV be it in vivo or invitro. In the present work two models are currently used to capture the kinetics of infection with influenza in vitro, are applied to study the kinetics of RSV infections in vitro. Literature data for in vitro RSV infections were digitally extracted from various publications and the parameters of the mathematical model were adjusted so as to best reproduce the quantity of virus over time reported in the publications. Through this process, and given the limited data available, we were able to confirm that both mathematical models can faithfully reproduce in vitro RSV infection kinetics. In addition approximate values were determined for some key viral replication parameters e.g. viral clearance rate, length of eclipse phase time to infection characterising RSV infection progression in vitro. This project lays the groundwork towards the development of an accurate mathematical model capable of capturing the details of an RSV infection in vitro.
This thesis represents the fulfillment of a requirement in order to obtain an BSc degree.